C program to Find all Roots of a Quadratic equation

This program accepts coefficients of a quadratic equation from the user and displays the roots (both real and complex roots depending upon the determinant).

The standard form of a quadratic equation is:

ax2 + bx + c = 0, where                       
a, b and c are real numbers and               
a ≠ 0                                         

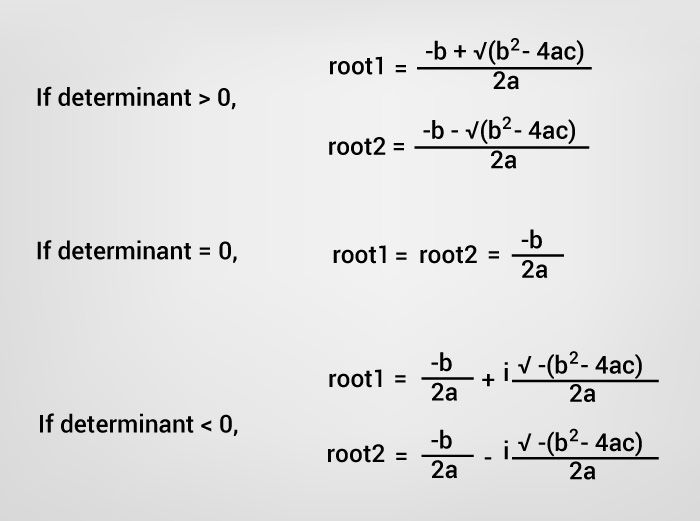
The term b2-4ac is known as the determinant of a quadratic equation. The determinant tells the nature of the roots.

• If determinant is greater than 0, the roots are real and different.
• If determinant is equal to 0, the roots are real and equal.
• If determinant is less than 0, the roots are complex and different.

Description

The C library function double sqrt(double x) returns the square root of x.

Declaration

Following is the declaration for sqrt() function.

double sqrt(double x)

Parameters

• x − This is the floating point value.

Return Value

This function returns the square root of x.

Example: Program to Find Roots of a Quadratic Equation

#include <stdio.h>
#include <math.h>

int main()
{
    double a, b, c, determinant, root1,root2, realPart, imaginaryPart;

    printf("Enter coefficients a, b and c: ");
    scanf("%lf %lf %lf",&a, &b, &c);

    determinant = b*b-4*a*c;

    // condition for real and different roots
    if (determinant > 0)
    {
    // sqrt() function returns square root
        root1 = (-b+sqrt(determinant))/(2*a);
        root2 = (-b-sqrt(determinant))/(2*a);

        printf("root1 = %.2lf and root2 = %.2lf",root1 , root2);
    }

    //condition for real and equal roots
    else if (determinant == 0)
    {
        root1 = root2 = -b/(2*a);

        printf("root1 = root2 = %.2lf;", root1);
    }

    // if roots are not real
    else
    {
        realPart = -b/(2*a);
        imaginaryPart = sqrt(-determinant)/(2*a);
        printf("root1 = %.2lf+%.2lfi and root2 = %.2f-%.2fi", realPart, imaginaryPart, realPart, imaginaryPart);
    }
    getch();
    return 0;
}

Output 

Enter coefficients a, b and c: 2.3                  
4                                                   
5.6                                                 
Roots are: -0.87+1.30i and -0.87-1.30i